Editor’s Note
We have already published Part I of this three-part article. In Part I, the author described the basics, including an ASBM’s trajectory, its guidance system, powered and glide flight paths, and other relevant details. In this Part, the article dwells upon reading the positioning of the missile system in flight, the problems faced with the earth not being a perfect sphere, and other errors concerning navigation, accelerometer, gyroscope and other miscellaneous influences.
The biggest problem in ballistic missile theory is to find the position of where I am now. Since it is a three-dimensional space, you have N, E and Down or X, Y and Z components in an orthogonal system. The calculations would have been very simple had the Earth been a perfect sphere and the value of acceleration due to gravity been constant. Unfortunately, the Earth is not a perfect sphere but an oblate spheroid. More so, when we view the Earth regarding Gravity values, it is nowhere close to even an oblate spheroid; it is more like a misshapen potato.
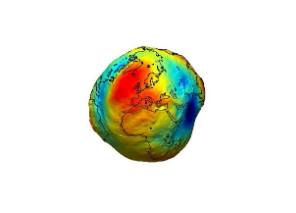
(Source: The European Space Agency)
It is so because the Earth’s mass is not evenly distributed. The measurement of gravity differs across the planet’s surface, which leads to something called free-air gravity anomalies, which need to be mapped. The Earth Gravitational Model 2008 gives an approximate gravitational map of the Earth, freely available as open source. Countries like the US have higher accuracy gravitation models of the Earth, but those are classified as having significant military purposes.
Now, gravity at the equator is approximately 9.76 m/s2, and at the poles, it is 9.832 m/s2. The rotation rate is 23 hours 56 minutes, and the rotation speed is 465 m/s or 1669 km/hr. The Earth also tilts to 23.4 degrees, oscillating between 22.1 to 24.5 degrees. We also have a Coriolis force. Therefore, the missile guidance system must compute all this to reach its target. We have an inertial navigation system on board, which was briefly discussed earlier in the article. Further amplifying the inertial navigation systems is essential to understand the subject clearly.
There are two types of inertial navigation systems—autonomous and assisted. These can be either gimballed or strapped down. The earlier systems were gimballed and mechanical because the technology of the day had not evolved sufficiently. They were bulky and super expensive to manufacture, being mechanical systems. The advantage was that there was no need to compensate for the missile’s motion. A strap-down system requires complex algorithms to pay as the system is physically fixed to the missile’s body.
The inertial navigation system on board a missile must be small in size and is called an Inertial Measurement Unit (IMU). The IMU has various grades depending on the type of usage. It ranges from instrumentation to tactical, navigation, and strategic. For a strategic missile, the accelerometer bias must be less than 1µg, and the gyroscopic bias needs to be less than 0.0001 degrees per hour. These are expensive to manufacture, and very few countries have the capability to make such strategic-grade IMUs. Even if they make them, the high cost and frequent replacements limit their utilisation of strategic nuclear missiles.
It is doubtful that any country would use such high-grade systems on a conventional ballistic missile. The ‘highly accurate’ IMU peddled by various companies has an accuracy of tactical grade at the best, and all of them require positional updates; otherwise, the drift rate errors are too high to be of any use.
Now, let us look at the frames of reference that a missile uses to hit the target accurately. Firstly, it has an inertial frame, which is fixed in space. Secondly is the earth-centred frame, which rotates with the earth. Thirdly is the Earth’s surface frame. Fourthly, it is the missile’s local frame. The equations of motion computed within these frames of reference are six Degree of Freedom (6DoF) equations because you have the three axes -X, Y and Z, and roll pitch and yaw. Computing 6DoF equations and controlling translation errors amongst the frames of references is no easy task.
More so, the accuracy of targeting is time-dependent. If used integral to the system, loosely coupledor tightly coupled, GPS or Baidu suffers from numerous errors; those that originate at the satellite, namely the satellite clocks, the selected availability and the accuracy of ephemeris data. GPS errors also originate in signal propagation or atmospheric refraction, causing atmospheric delays.
Then, some errors originate at the receiver, namely multipath and receiver clock accuracy. At this point, a bit of Einsteinian relativity also comes into play. Various effects that make a difference are gravitational redshift, time dilation, Sagnac effect, inter-satellite links, earth oblateness, curvature delay, Shapiro delay, tidal potential and the Lense-Thirring effect. All these add to GPS errors. So, next time you think GPS is very accurate, it is not, especially regarding ballistic missile targeting.
If we were to summarise the sources of ballistic missile errors, they could be clubbed under navigation, accelerometer, gyroscope, and miscellaneous. There are 24 basic errors, which actually translate into computing over 300 global parameters. The majority of these calculations are completed prior to the missile’s launch, and the balance is completed while in flight.
Now, let us come to the re-entry phase. According to open-source information and those fabulous YouTube videos you would have seen, a ballistic missile warhead enters the atmosphere and starts revolving at two revolutions per second. The missile acceleration reaches 120 G, the missile velocity is eight km per second, and the re-entry time is two minutes. The videos would have you believe that the cone-shaped warhead revolves exactly and hits the target with pinpoint accuracy. Not true!
On entry, a warhead is subjected to intense heat due to atmospheric friction. The warhead needs a protective shield to protect it and the guidance system. In the olden days, the heat shield was a heat sink, a heavy layer of aluminium, steel, or beryllium alloy that, due to aerodynamics, was blunt-shaped. These wobbled on the way down, as described by Graham Spinardi in his excellent book From Polaris to Trident, resulting in a large Circular Error Probability (CEP).
All ballistic missile warheads now have a carbon-carbon ablative shield that burns off. Though the weave pattern does control the wobble to a large extent, weather encountered on the way down still induces a certain amount of wobble and error in hitting the target. The errors, however, are manageable as the target is a stationary fixed target. The launch position is also fixed. A moving launch platform such as a ship, a submarine, or an aircraft introduces even more complications, as the 6DoF equations of the platform’s motion also need to be calculated.
Rear Admiral Ajay V Bhave (Retd)
(Note: In the concluding Part III, the focus will shift to an early example of a ballistic missile that was used as a template by the Chinese to create their famed DF-21D ASBM and the possible mechanics of the ‘kill chain’.)








































